Examples
Every statement of percent involves three numbers. For example,
8 is 50% of 16.
8 is called the Amount. 50% is the Percent. 16 is called the Base. The Base always follows "of." What you see above is the standard form of any statement of percent.
The Amount is some Percent of the Base.
In a percent problem, we are given two of those numbers and we are asked to find the third. We have already seen how to solve any percent problem with a calculator. The same procedures apply in a written calculation, in which we would typically change the percent to a decimal.
We saw in Lesson 4 how to take 1% and 10% of a number simply by placing the decimal point. Those should be basic skills. What is more, from 1% we can calculate 2%, 3%, and so on. While from 10% we can easily calculate 20%, 30% and any multiple of 10%.
Examples
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
32% of 100 is 32. 87.9% of 100 is 87.9. 416% of 100 is 416. (percent is an abbreviation for the Latin per centum, which means for each 100). (Per means for each.) A percent is a number of hundredths.
Example 1. A store
paid $100 for a jacket. It then raised the selling price by 28%. But a
week later it reduced that price by 10%. What was the final selling
price?
Solution. 28% of $100 is $28. So the selling price became $128.
To subtract $12.80 from $128, round it off to $13:
$128 − $13 = $115, plus $.20 is $115.20.
That was the final selling price.
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
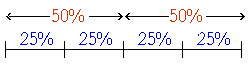
25% is half of 50%.
Example 2. How much is 25% of 60?
Answer. Half of 60 is 30. Half of 30 is 15.
Example 3. How much is 25% of 180?
Answer. Half of 180 is 90. Half of 90 is 45.
Example 4. How much is 25% of 112?
Answer. Half of 112 = Half of 100 + Half of 12 = 56.
Answer. Half of 56 = Half of 50 + Half of 6 = 25 + 3 = 28.
Example 5. How much is 25% of $9.60?
Answer. Half of $9.60 = $4.50 + $.30 = $4.80. Half of $4.80 = $2.40
Example 6. Eighths. How much is 37.5% of $600?
Answer. Upon recognizing that 37.5% means three eighths
First, a quarter of $600 is half of $300: $150. And an eighth is half of a quarter: $75. Therefore three eighths are three times $75: $225.
Equivalently, one quarter -- $150 -- is two eighths. Therefore, three
eighths will be one quarter plus half of one quarter: $150 + $75 =
$225. |
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Example 7. How much is 15% of $70??
Example 8. If you tip at the rate of 15%, and the bill is $40, how much do you leave?
In general:
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Example 9. Percent to a decimal. How much is 11% of $420?
Solution 1. 11% is 11 hundredths, which we can represent as the decimal .11. Therefore,
11% of $420 = .11 × 420.
Now,
Therefore, on separating two decimal digits,
11% of $420 = $46.20.
Solution 2. More simply, since 11% = 10% + 1%, then
Example 10. How much is 30% of $48?
Solution. The
student should realize that 30% is simply three times 10% and so will
always involve multiplication by 3. (Its decimal is .3.)
Now, 10% of $48 is $4.80. Therefore, 30% is
See Lesson 9, The distributive property of multiplication, Examples 5 and 6.
Example 11. How much is 40% of $90?
Solution. 4 times 9 is 36. 40% of $90 is $36.
Example 12. Vanessa is about to withdraw $3000 from her retirement account. But 20% will be withheld for taxes. How much will she actually receive?
Solution. Since 20% will be withheld, she will receive 80%. (The whole is 100%.) 80% of $3000 is
.8 × 3000 = 2400.
( The 0 of .80 is unnecessary.)
She will receive $2400.
Example 13. How much is 80% of $45?
Solution 1. If we multiplied by .8, the answer would have one decimal digit.
For it is easy to do 4.5 × 8, that is -- Four and a half times 8.
"Four times 8 is 32. Half of 8 is 4. 32 plus 4 is 36."
80% of $45 is $36.
Solution 2. 80% means four fifths; 45 has an exact fifth part; therefore we can reason as follows:
One fifth of 45 is 9. Therefore four fifths are four 9's -- 36.
Example 14. How much is 75% of 108?
Answer. We could write 75% as the decimal .75, and then multiply
.75 × 108.
However, 75% is three quarters. Therefore we could calculate
Three quarters of 108.
That is not difficult if we decompose 108 into 100 + 8.
Example 15. How much is 18.9% of $314?
Answer. Use your calculator
To do a problem in writing, then, we must express
the percent either as a decimal or a fraction. As for expressing a
percent as a fraction:
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Examples 16.
In addition, the student should know:
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Example 17. How much is 250% of 32?
Answer. 250% = 2½.
Example 18. How much is 37½% of $40?
three eighths are 3 × $5 = $15.)
can decompose it into multiples of 3 as follows:
720 = 600 + 120.
A third of 600 is 200.
A third of 120 is 40.
Therefore a third of 720 is 240.
Solution. To find two thirds, we must first find one third, and then multiply by 2. Press
See
This is approximately $50.85.
The standard textbook method for finding a percent of a number,
has been to change the percent to a decimal, and multiply. And so to
find 24% of $412, we are taught to change 24% to the decimal .24 , and multiply times 412.
But is anyone with a calculator
going to do that these days? And aren't there more important things to
learn about percent? Like how to take 25% of $412 without writing
anything! Take half of 50%, which is $206. 25% is $103.
24% of $412 will then be 25% − 1%:
Example 21. $36 is 4% of how much?
Answer. Here, the Base is missing, the number that follows.
Video Help
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||

No comments:
Post a Comment