Solving Proportions (4-4)

Solving Proportions

Vocabulary

Cross Product- The product of one numerator and the opposite denominator in a pair of equivalent
                        fractions.

Examples
Solving proportions is simply a matter of stating the ratios as fractions, setting the two fractions equal to each other, cross-multiplying, and solving the resulting equation. You'll probably start out by just solving proportions, like this:

• Find the unknown value in the proportion:  2 : x = 3 : 9.
2 : x = 3 : 9
First, I convert the colon-based odds-notation ratios to fractional form:

.
Then I solve the proportion:


9(2) = x(3)
18 = 3x
6 = x

• Find the unknown value in the proportion:  (2x + 1) : 2 = (x + 2) : 5
(2x + 1) : 2 = (x + 2) : 5

	ADVERTISEMENT

First, I convert the colon-based odds-notation ratios to fractional form:

Then I solve the proportion:


5(2x + 1)  =  2(x + 2)
10x + 5  =  2x + 4
8x  =  –1
x = ^–1/₈

---

Once you've solved a few proportions, you'll likely then move into word problems where you'll first have to invent the proportion, extracting it from the word problem, before solving it.

• If twelve inches correspond to 30.48 centimeters, how many centimeters are there in thirty inches?
I will set up my ratios with "inches" on top, and will use "c" to stand for the number of centimeters for which they've asked me.



12c = (30)(30.48)
12c = 914.4
c = 76.2

Thirty inches corresponds to 76.2 cm.

I could have used any letter I liked for my variable. I chose to use "c" because this will help me to remember what the variable is representing. An "x" would only tell me that I'm looking for "some unknown value"; a "c" can remind me that I'm looking for "centimeters". Warning: Don't fall into the trap of feeling like you "have" to use "x" for everything. You can use whatever you find most helpful.

• A metal bar ten feet long weighs 128 pounds. What is the weight of a similar bar that is two feet four inches long?

First, I'll need to convert the "two feet four inches" into a feet-only measurement. Since four inches is four-twelfths, or one-third, of a foot, then:

2 feet + 4 inches = 2 feet +  ¹/₃ feet  =  ⁷/₃ feet
I will set up my ratios with the length values on top, set up my proportion, and then solve for the required weight value:



Since this is a "real world" word problem, I should probably round or decimalize my exact fractional solution to get a practical "real world" sort of number.

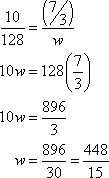
The bar will weigh ⁴⁴⁸/₁₅ , or about 29.87, pounds.

• The tax on a property with an assessed value of $70 000 is $1 100. What is the assessed value of a property if the tax is $1 400?
I will set up my ratios with the assessed valuation on top, and I will use "v" to stand for the value that I need to find. Then:



98 000 000   = 1 100v
89 090.909 090 9... = v

Since the solution is a dollars-and-cents value, I need to round the final answer to two decimal places: Copyright © Elizabeth Stapel 2001-2011 All Rights Reserved

The assessed value is $89 090.91.

• One piece of pipe 21 meters long is to be cut into two pieces, with the lengths of the pieces being in a 2 : 5 ratio. What are the lengths of the pieces?

I'll label the length of the short piece as "x". Then the long piece, being the total piece less what was cut off for the short piece, must have a length of 21 – x.

(short piece) : (long piece):  2 : 5  =  x : (21 – x)

2(21 – x) = 5x
42 – 2x = 5x
42 = 7x
6 = x
Referring back to my set-up for my equation, I see that I defined "x" to stand for the length of the shorter piece. Then the length of the longer piece is given by:

21 – x = 21 – 6 = 15
Now that I've found both required values, I can give my answer:

The two pieces have lengths of 6 meters and 15 meters.

In the last exercise above, if I had not defined what I was using "x" to stand for, I could easily have overlooked the fact that "x = 6" was not the answer the exercise was wanting. Try always to clearly define and label your variables. Also, be sure to go back and re-check the word problem for what it actually wants. This exercise did not ask me to find "the value of a variable" or "the length of the shorter piece". By re-checking the original exercise, I was able to provide an appropriate response, being the lengths of the two pieces, including the correct units ("meters"). 
 http://www.purplemath.com/modules/ratio4.htm

 Video Help

 https://www.khanacademy.org/math/algebra-basics/core-algebra-linear-equations-inequalities/ratios-core-algebra/v/find-an-unknown-in-a-proportion

 https://www.youtube.com/watch?v=he8Gl03cZHg

 https://www.youtube.com/watch?v=kAW-JB1IVxA